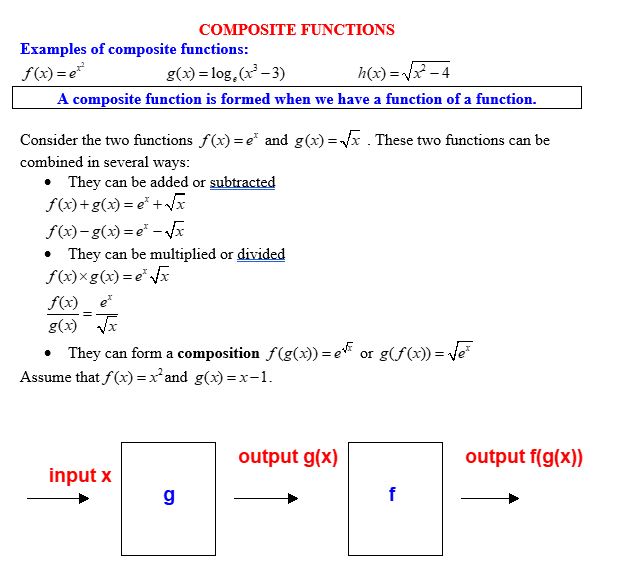
Composite functions are a fundamental concept in mathematics, often used in algebra, calculus, and other branches of math. They involve combining two or more functions to create a new function. This set of notes and examples provides a concise introduction to composite functions, explaining the concept and providing illustrative examples.
$0.00
Definition (Composite Function): A composite function, denoted as (f ∘ g)(x), is formed by applying one function, g(x), to the result of another function, f(x). The composite function (f ∘ g)(x) is read as “f of g of x” or “f composed with g of x.”
Notation:
- (f ∘ g)(x) = f(g(x))
Evaluating Composite Functions: To evaluate a composite function (f ∘ g)(x).
These notes and examples provide a clear understanding of composite functions, their notation, and how to evaluate them by substituting one function into another. Students can use these concepts to solve more complex mathematical problems and understand the composition of functions in various mathematical contexts.